Tangents and Secants to a Circle

Question 1.
Fill in the blanks.

• i) A tangent to a circle intersects it in ——— point(s). (one)
• ii) A line intersecting a circle in two points is called a ———. (secant)
• iii) The number of tangents drawn at the end of the diameter is ———. (two)
• iv) The common point of a tangent to a circle and the circle is called ———. (point of contact)
• v) We can draw ——— tangents to a given circle. (infinite)

Question 2.
A tangent PQ at a point P of a circle of radius 5 cm meets a line through the centre O at a point Q so that OQ = 12 cm. Find length of PQ.

Answer:
Given: A circle with centre O and radius OP = 5 cm

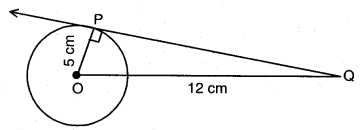
We know that ∠OPQ = 90°
Hence in △OPQ
OQ²= OP²+ PQ²
[∵ hypotenuse²= Adj. side²+ Opp. side²]
12²= 5²+ PQ²
∴ PQ²= 144 - 25 .
PQ²= 119
PQ = √119

Question 3.
Draw a circle and two lines parallel to a given line such that one is a tangent and the other, a secant to the circle.

Answer:

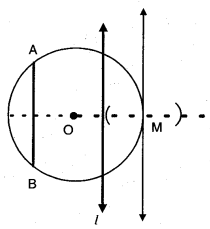
Steps:

1. Draw a circle with some radius.
2. Draw a chord of the circle.
3. Draw a line parallel to the chord intersecting the circle at two distinct points.
4. This is secant of the circle (l).
5. Draw another line parallel to the chord, just touching the circle at one point (M). This is a tangent of the circle.

Question 4.
Calculate the length of tangent from a point 15 cm. away from the centre of a circle of radius 9 cm.

Answer:

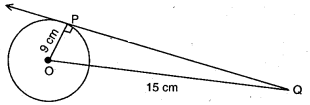
Given: A circle with radius OP = 9 cm
A tangent PQ from a point Q at a distance of 15 cm from the centre, i.e., OQ =15 cm
Now in △POQ, ∠P = 90°
OP²+ PQ²- OQ²
9²+ PQ²= 15²
PQ²= 15²- 9²
PQ²= 144
∴ PQ = √144 = 12 cm.
Hence the length of the tangent =12 cm.

Question 5.
Prove that the tangents to a circle at the end points of a diameter are parallel.

Answer:
A circle with a diameter AB.
PQ is a tangent drawn at A and RS is a tangent drawn at B.
R.T.P: PQ ‖ RS.
Proof: Let ‘O’ be the centre of the circle then OA is radius and PQ is a tangent.
∴ OA ⊥ PQ ……….(1)
[∵ a tangent drawn at the end point of the radius is perpendicular to the radius]
Similarly, OB ⊥ RS ……….(2)
[∵ a tangent drawn at the end point of the radius is perpendicular to the radius]
But, OA and OB are the parts of AB.
i.e., AB ⊥ PQ and AB ⊥ RS.
∴ PQ ‖ RS.

O is the centre, PQ is a tangent drawn at A.
∠OAQ = 90°
Similarly, ∠OBS = 90°
∠OAQ + ∠OBS = 90° + 90° = 180°
∴ PQ ‖ RS.
[∵ Sum of the consecutive interior angles is 180°, hence lines are parallel]

Question 1.
Choose the correct answer and give justification for each.

(i) The angle between a tangent to a circle and the radius drawn at the point of contact is

• a) 60°
• b) 30°
• c) 45°
• d) 90°

Answer: [ d ]

If radius is not perpendicular to the tangent, the tangent must be a secant i.e., 90°.

(ii) From a point Q, the length of the tangent to a circle is 24 cm. and the distance of Q from the centre is 25 cm. The radius of the circle is

• a) 7 cm
• b) 12 cm
• c) 15 cm
• d) 24.5 cm

Answer: [ a ]

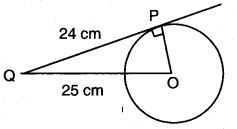
O - centre of the circle
OP - a circle radius = ?
OQ = 25 cm
PQ = 24 cm
OQ²= OP²+ PQ²
[∵ hypotenuse²= Adj. side² + Opp. side²]
25²= OP²+ 24²
OP²= 625 - 576
OP²= 49
OP = √49 = 7 cm.

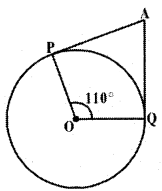
iii) If AP and AQ are the two tangents a circle with centre O, so that ∠POQ = 110°. Then ∠PAQ is equal to

a) 60°
b) 70°
c) 80°
d) 90°
Answer: [ b ]
In □ OPAQ,
∠OPA = ∠OQA = 90°
∠POQ = 110°
∴ ∠O + ∠P + ∠A + ∠Q = 360°
⇒ 90° + 90° + 110° + ∠PAQ - 360°
⇒ ∠PAQ = 360° - 290° = 70°

iv) If tangents PA and PB from a point P to a circle with centre O are inclined to each other at angle of 80°, then ∠POA is equal to

• a) 50°
• b) 60°
• c) 70°
• d) 80°

Answer: [None]

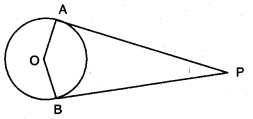
If ∠APB = 80°
then ∠AOB = 180° - 80° = 100°
[∴ ∠A + ∠B = 90° + 90° = 180°]

v) In the figure XY and XV are two parallel tangents to a circle with centre O and another tangent AB with point of contact C intersecting XY at A and XV at B then ∠AOB =

• a) 80°
• b) 100°
• c) 90°
• d) 60°

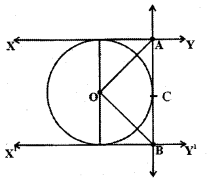
Answer: [ c ]

Question 2.
Two concentric circles of radii 5 cm and 3 cm are drawn. Find the length of the chord of the larger circle which touches the smaller circle.

Answer:
Given: Two circles of radii 3 cm and 5 cm with common centre.

Let AB be a tangent to the inner/small circle and chord to the larger circle.
Let ‘P’ be the point of contact.
Construction: Join OP and OB.
In △OPB ;
∠OPB = 90°
[radius is perpendicular to the tangent]
OP = 3cm OB = 5 cm
Now, OB²= OP²+ PB²
[hypotenuse²= Adj. side²+ Opp. side², Pythagoras theorem]
5²= 3²+ PB²
PB²= 25 - 9 = 16
∴ PB = √l6 = 4cm.
Now, AB = 2 × PB
[∵ The perpendicular drawn from the centre of the circle to a chord, bisects it]
AB = 2 × 4 = 8 cm.
∴ The length of the chord of the larger circle which touches the smaller circle is 8 cm.

Question 3.
Prove that the parallelogram circumscribing a circle is a rhombus.

Answer:

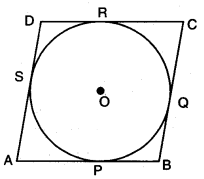
Given: A circle with centre ‘O’.
A parallelogram ABCD, circumscribing the given circle.
Let P, Q, R, S be the points of contact.
Required to prove: □ ABCD is a rhombus.
Proof: AP = AS …….. (1)
[∵ tangents drawn from an external point to a circle are equal]
BP = BQ ……. (2)
CR = CQ ……. (3)
DR = DS ……. (4)
Adding (1), (2), (3) and (4) we get
AP + BP + CR + DR = AS + BQ + CQ + DS
(AP + BP) + (CR + DR) = (AS + DS) + (BQ + CQ)
AB + DC = AD + BC
AB + AB = AD + AD
[∵ Opposite sides of a parallelogram are equal]
2AB = 2AD
AB = AD
Hence, AB = CD and AD = BC [∵ Opposite sides of a parallelogram]
∴ AB = BC = CD = AD
Thus □ ABCD is a rhombus (Q.E.D.)

Question 4.
A triangle ABC is drawn to circumscribe a circle of radius 3 cm such that the segments BD and DC into which BC is divided by the point of contact D are of length 9 cm. and 3 cm. respectively (See below figure). Find the sides AB and AC.

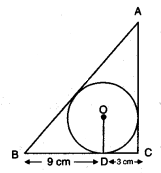
Answer:
The given figure can also be drawn as

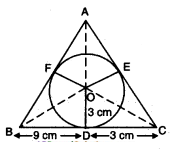
Given: Let △ABC be the given triangle circumscribing the given circle with centre ‘O’ and radius 3 cm.
i.e., the circle touches the sides BC, CA and AB at D, E, F respectively.
It is given that BD = 9 cm
CD = 3 cm

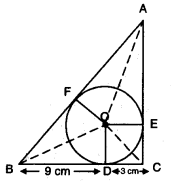
∵ Lengths of two tangents drawn from an external point to a circle are equal.
∴ BF = BD = 9 cm
CD = CE = 3 cm
AF = AE = x cm say
∴ The sides of die triangle are
12 cm, (9 + x) cm, (3 + x) cm
Perimeter = 2S = 12 + 9 + x + 3 + x
⇒ 2S = 24 + 2x
or S = 12 + x
S - a = 12 + x - 12 = x
S - b = 12 + x - 3 - x = 9
S - c = 12 + x - 9 - x = 3
∴ Area of the triangle

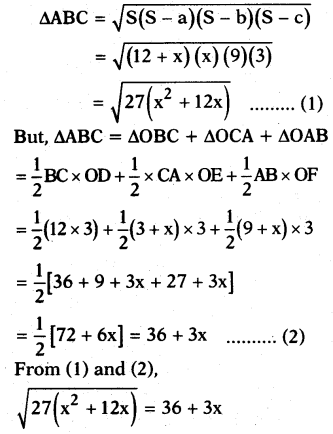
Squaring on both sides we get,
27 (x²+ 12x) = (36 + 3x)²
27x²+ 324x = 1296 + 9x²+ 216x
⇒ 18x²+ 108x- 1296 = 0
⇒ x²+ 6x - 72 = 0
⇒ x²+ 12x - 6x - 72 = 0
⇒ x (x + 12) - 6 (x + 12) = 0
⇒ (x - 6) (x + 12) = 0
⇒ x = 6 or - 12
But ‘x’ can’t be negative hence, x = 6
∴ AB = 9 + 6 = 15 cm
AC = 3 + 6 = 9 cm.

Question 5.
Draw a circle of radius 6 cm. From a point 10 cm away from its centre, construct the pair of tangents to the circle and measure their lengths. Verify by using Pythagoras Theorem.

Answer:
Steps of construction:

1. Draw a circle with centre ‘O’ and radius 6 cm.
2. Take a point P outside the circle such that OP =10 cm. Join OP.
3. Draw the perpendicular bisector to OP which bisects it at M.
4. Taking M as centre and PM or MO as radius draw a circle. Let the circle intersects the given circle at A and B.
5. Join P to A and B.
6. PA and PB are the required tan¬gents of lengths 8 cm each.

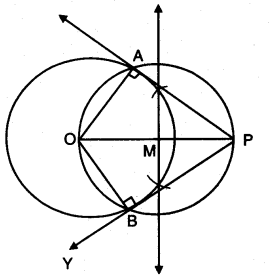
Proof: In △OAP
OA²+ AP²= 6²+ 8²
= 36 + 64 = 100
OP²= 10²= 100
∴ OA²+ AP²= OP²
Hence AP is a tangent.
Similarly BP is a tangent.

Question 6.
Construct, a tangent to a circle of radius 4 cm from a point on the concentric circle of radius 6 cm and measure its length. Also verify the measurement by actual calculation.

Answer:

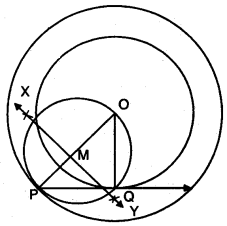
Steps of construction:

1. Draw two concentric circles with centre ‘O’ and radii 4 cm and 6 cm.
2. Take a point ‘P’ on larger circle and join O, P.
3. Draw the perpendicular bisector of OP which intersects it at M.
4. Taking M as centre and PM or MO as radius draw a circle which intersects smaller circle at Q.
5. Join PQ, which is a tangent to the smaller circle.

Question 7.
Draw a circle with the help of a bangle, take a point outside the circle. Con-struct the pair of tangents from this point to the circle measure them. Write conclusion.

Answer:

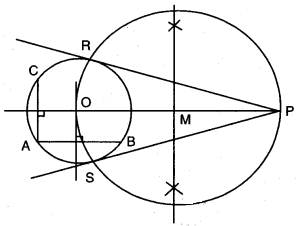
Steps of construction:

1. Draw a circle with the help of a bangle.
2. Draw two chords AB and AC. Perpendicular bisectors of AB and AC meets at ‘O’ which is the centre of the circle.
3. Taking an outside point P, join OP.
4. Let M be the midpoint of OP. Taking M as centre OM as radius, draw a circle which intersects the given circle at R and S. Join PR, PS which are the required tangents.

Conclusion: Tangents drawn from an external point to a circle are equal.

Question 8.
In a right triangle ABC, a circle with a side AB as diameter is drawn to intersect the hypotenuse AC in P. Prove that the tangent to the circle at P bisects the side BC.

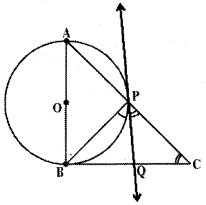
Answer:
Let ABC be a right triangle right angled at P.
Consider a circle with diametere AB.
From the figure, the tangent to the circle at B meets BC in Q.
Now QB and QP are two tangents to the circle from the same point P.
QB = QP …….. (1)
Also, ∠QPC = ∠QCP
∴ PQ = QC (2)
From (1) and (2);
QB = QC Hence proved.

Question 9.
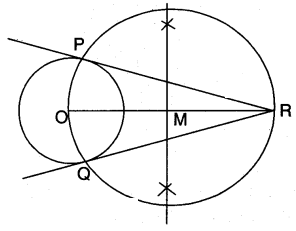
Draw a tangent to a given circle with center O from a point ‘R’ outside the circle. How many tangents can be drawn to the circle from that point? [Hint: The distance of two points to the point of contact is the same.

Answer:
Only two tangents can be drawn from a given point outside the circle.

Question 1.
A chord of a circle of radius 10 cm. subtends a right angle at the centre. Find the area of the corresponding: (use π = 3.14)

i) Minor segment ii) Major segment

Answer:

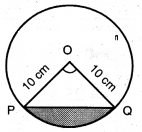
Angle subtended by the chord = 90° Radius of the circle = 10 cm
Area of the minor segment = Area of the sector POQ - Area of △POQ
Area of the sector = x/360 × πr²
90/360 × 3.14 × 10 × 10 = 78.5
Area of the triangle =12× base × height
= 1/2 × 10 × 10 = 50
∴ Area of the minor segment = 78.5 - 50 = 28.5 cm²
Area of the major segment = Area of the circle - Area of the minor segment
= 3.14 × 10 × 10 - 28.5
= 314 - 28.5 cm²
= 285.5 cm²

Question 2.
A chord of a circle of radius 12 cm. subtends an angle of 120° at the centre. Find the area of the corresponding minor segment of the circle.
(use π = 3.14 and √3 = 1.732)

Answer:
Radius of the circle r = 12 cm.
Area of the sector = x/360 × πr²
Here, x = 120°

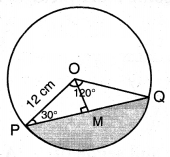
120/360 × 3.14 × 12 × 12 = 150.72
Drop a perpendicular from ‘O’ to the chord PQ.
△OPM = △OQM [∵ OP = OQ ∠P = ∠Q; angles opp. to equal sides OP & OQ; ∠OMP = ∠OMQ by A.A.S]
∴ △OPQ = △OPM + △OQM = 2 . △OPM
Area of △OPM = 1/2 × PM × OM

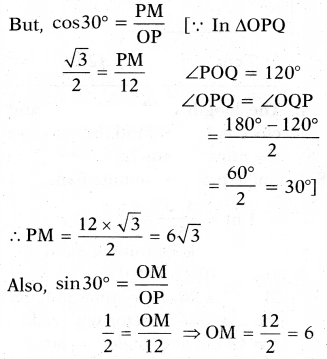
= 18 × 1.732 = 31.176 cm
∴ △OPQ = 2 × 31.176 = 62.352 cm²
∴ Area of the minor segment

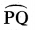
= (Area of the sector) - (Area of the △OPQ)
= 150.72 - 62.352 = 88.368 cm²

Question 3.
A car has two wipers which do not overlap. Each wiper has a blade of length 25 cm. sweeping through an angle of 115°. Find the total area cleaned at each sweep of the blades. (use π =22/7)

Answer:
Angle made by the each blade = 115°
Total area swept by two blades
= Area of the sector with radius 25 cm and angle 115°+ 115° = 230°
= Area of the sector = x/360 × πr²
=230/360 × 22/7 × 25 × 25
= 1254.96
≃ 1255 cm²

Question 4.
Find the area of the shaded region in figure, where ABCD is a square of side 10 cm. and semicircles are drawn with each side of the square as diameter (use π = 3.14).

Answer:
Let us mark the four unshaded regions as I, II, III and IV.

Area of I + Area of II
= Area of ABCD - Areas of two semicircles with radius 5 cm
= 10 × 10 - 2 × 1/2 × π × 5²
= 100 - 3.14 × 25
= 100 - 78.5 = 21.5 cm²
Similarly, Area of II + Area of IV = 21.5 cm²
So, area of the shaded region = Area of ABCD - Area of unshaded region
= 100 - 2 × 21.5 = 100 - 43 = 57 cm²

Question 5.
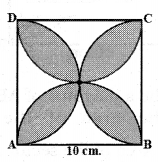
Find the area of the shaded region in figure, if ABCD is a square of side 7 cm. and APD and BPC are semicircles. (use π = 22/7)

Answer:
Given,
ABCD is a square of side 7 cm.
Area of the shaded region = Area of ABCD - Area of two semicircles with radius 7/2 = 3.5 cm
APD and BPC are semicircles.
= 7 × 7 - 2 × 1/2 × 22/7 × 3.5 × 3.5
= 49 - 38.5
= 10.5 cm²
∴ Area of shaded region = 10.5 cm

Question 6.
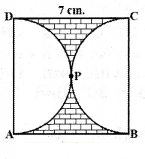
In figure, OACB is a quadrant of a circle with centre O and radius 3.5 cm. If OD = 2 cm., find the area of the shaded region, (use π = 22/7).

Answer:
Given, OACB is a quadrant of a Circle.
Radius = 3.5 cm; OD = 2 cm.
Area of the shaded region = Area of the sector - Area of △BOD

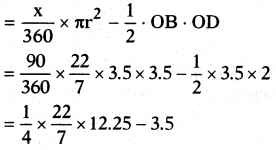
= 9.625 - 3.5 = 6.125 cm²
∴ Area of shaded region = 6.125 cm².

Question 7.
AB and CD are respectively arcs of two concentric circles of radii 21 cm. and 7 cm. with centre O (See figure). If ∠AOB = 30°, find the area of the shaded region. (use π = 22/7).

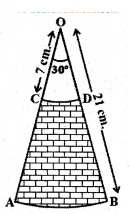
Answer:
Given, AB and CD are the arcs of two concentric circles.
Radii of circles = 21 cm and 7 cm and ∠AOB = 30°
We know that,
Area of the sector = x/360 × πr²
Area of the shaded region = Area of the OAB - Area of OCD

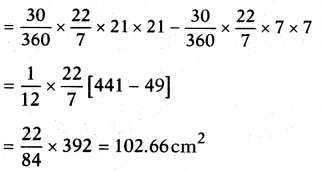
∴ Area of shaded region = 102.66 cm²

Question 8.
Calculate the area of the designed region in figure, common between the two quadrants of the circles of radius 10 cm each, {use π = 3.14)

Answer:
Mark two points P, Q on the either arcs.
Let BD be a diagonal of ABCD
Now the area of the segment

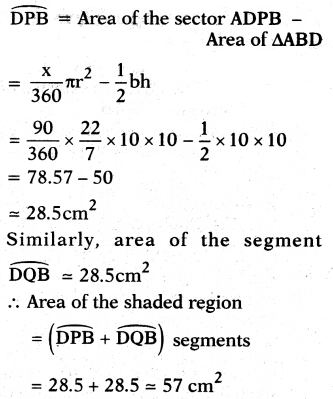
= 28.5 + 28.5 = 57 cm²

Side of the square = 10 cm
Area of the square = side × side
= 10 × 10 = 100 cm²
Area of two sectors with centres A and C and radius 10 cm.
= 2 × ?r²/360 × x = 2 × x/360 × 22/7 × 10 × 10
=1100/7
= 157.14 cm²
∴ Designed area is common to both the sectors,
∴ Area of design = Area of both sectors - Area of square
= 157 - 100 = 57 cm²
(or)
1100/7 - 100 = 1100?700/7
=400/7
= 57.1 cm²